When the neutrons slow down in a medium with resonance absorption present, the smooth weighting function discussed above will be modified. Dips will appear in the flux corresponding to resonance peaks, and sometimes sharp peaks will occur in the flux corresponding to deep minima in the cross section, or "windows." The products of cross section and flux that appear in the definitions of the multigroup constants will clearly be reduced (self shielded) when the dips are large. 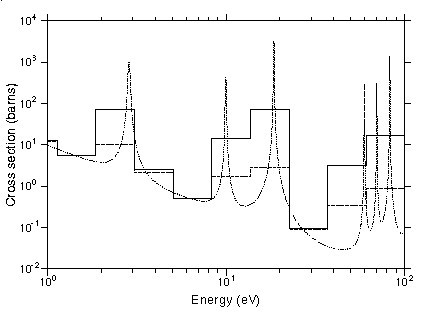The classical method for handling self shielding in multigroup codes is the Bondarenko model. 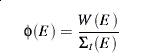For narrow resonances in large systems, the flux takes the form: The denominator contains the total macroscopic cross section for the material. To obtain the part of the flux that provides self shielding for isotope i, it is assumed that all the other isotopes can be represented with a constant "background cross section" called σ0. 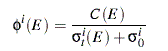Therefore, The qualitative behavior of this equation is easy to understand. If &simga;0 is larger than the tallest peaked in &simga;t, the weighting flux is approximately proportional to the smooth weighting function C(E). This is called infinite dilution; the cross section in the material of interest has little or no effect on the flux. On the other hand, if σ0 is small with respect to σt, the weighting flux will have large dips at the locations of the peaks in σt, and a large self-shielding effect will be expected, The following input deck will prepare self shielded cross sections for three temperatures and seven values of σ0 for Pu-238 from ENDF/B-VI. To understand the deck, compare it with the GROUPR input instructions. A convenient way to do this is to bring the instructions up in a separate window using the right mouse button.

      groupr
      21 22 0 23
      9434 6 0 4 0 3 7 1
      '94-pu-238'/
      300. 900. 2100.
      1.e10 1.e5 1.e4 1000. 100. 10. 1
      .1 0.025 0.8208e06 1.4e06
      3 1 'total'/
      3 2 'elastic'/
      3 18 'fission'/
      3 102 'capture'/
      0/
      3 1 'total'/
      3 2 'elastic'/
      3 18 'fission'/
      3 102 'capture'/
      0/
      3 1 'total'/
      3 2 'elastic'/
      3 18 'fission'/
      3 102 'capture'/
      0/
      0/

     group constants at t=3.000E+02 deg k 
     for mf  3 and mt102 capture
    
     enrgy  group constants vs sigma zero
     group  infinity  ... 1.000E+03  1.000E+02  1.000E+01  1.000E+00

       1    3.494E+02 ... 2.927E+02  1.995E+02  1.487E+02  1.396E+02
       2    1.254E+01 ... 1.253E+01  1.246E+01  1.227E+01  1.219E+01
       3    5.580E+00 ... 5.578E+00  5.565E+00  5.521E+00  5.495E+00
       4    7.307E+01 ... 5.052E+01  2.244E+01  1.204E+01  1.029E+01
       5    2.510E+00 ... 2.500E+00  2.434E+00  2.227E+00  2.115E+00
       6    5.200E-01 ... 5.200E-01  5.198E-01  5.189E-01  5.181E-01
       7    1.425E+01 ... 1.099E+01  4.944E+00  2.225E+00  1.743E+00
       8    7.344E+01 ... 2.738E+01  8.348E+00  3.696E+00  2.899E+00
       9    9.300E-02 ... 9.297E-02  9.275E-02  9.174E-02  9.083E-02
      10    3.183E+00 ... 2.645E+00  1.262E+00  4.791E-01  3.409E-01
      11    1.694E+01 ... 8.739E+00  2.943E+00  1.165E+00  8.858E-01
      12    2.627E+01 ... 1.489E+01  5.083E+00  1.979E+00  1.488E+00
      13    2.337E+01 ... 1.254E+01  4.472E+00  1.981E+00  1.592E+00
      14    1.348E+01 ... 8.083E+00  3.312E+00  1.459E+00  1.123E+00
      15    1.081E+01 ... 6.994E+00  3.060E+00  1.373E+00  1.056E+00
      16    7.869E+00 ... 5.761E+00  2.772E+00  1.277E+00  9.828E-01
      17    6.324E+00 ... 4.939E+00  2.560E+00  1.219E+00  9.415E-01
      18    5.154E+00 ... 4.223E+00  2.352E+00  1.165E+00  9.056E-01
      19    4.195E+00 ... 3.579E+00  2.141E+00  1.109E+00  8.704E-01

And finally, we show a plot of the PENDF cross section for capture (dotted), the infinitely-dilute capture (solid), and the self-shielded capture for a background cross section of 1 barn (dashed).